Recurring Decimal to Vulgar Fraction

In this class, We discuss Recurring Decimal to Vulgar Fraction.

For Complete YouTube Video: Click Here

The reader should have prior knowledge of vulgar fractions. Click Here.

Recurring Decimal: if in a decimal fraction a digit or a set of digits are repeated continuously, call recurring Decimal.

Example 0.555, 3.142857142857

In 0.555, digit five is repeated endlessly.

In 3.142857142857, the digits 142857 are repeated.

If a single digit is repeated we represent it with dot

The below diagram shows the example.

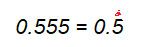
If a set of digits are repeated, we place a bar.

The below diagram shows the example.

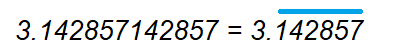
Pure Recurring Decimal:

If all digits after Decimal are repeating, we call pure recurring Decimal.

The below diagram shows the examples.

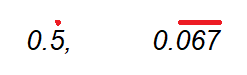
Convert pure recurring to a vulgar fraction

Take the repeated digits only once in the numerator.

Take as many 9s as the number of repeating digits in the denominator.

Example:

The below diagram shows the examples.

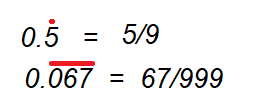
0.067 can be written as 67/999

numerator, we write the digits after Decimal, i.e., 067.

zero before the integer is no value. So we write 67 in the numerator.

In the denominator, we write three 9’s. Because we have three digits after the Decimal in 0.067.

Mixed Recurring Decimal:

A decimal in which some digits are not repeated, and some are repeated, we call a mixed recurring Decimal.

The below diagram shows the example of mixed recurring Decimals.

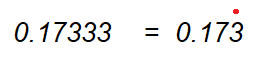
Digit Seventeen is not repeated, and digit three is repeated.

Convert Mixed Recurring Decimal to Vulgar Fraction:

In the numerator

take the difference between the number formed by all the digits after Decimal and those not repeating.

denominator

Take as many 9s as the number of repeating digits followed by as many zeroes as the number of non-repeating digits.

The below diagram shows the example.

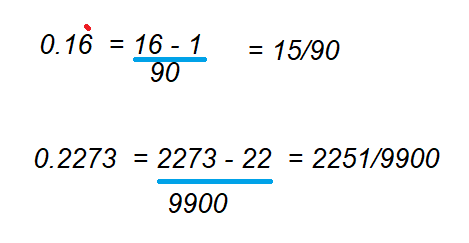
In the numerator, we take 16 – 1.

digits after Decimal are 16.

subtract 16 with the non-repeating digits.

The non-repeating digits are 1.

In the denominator, we consider 90.

The number of repeating digits is one, so one nine.

The number of non-repeating digits is one. So one zero.

We get 90 in the denominator.

In the second example

The numerator value 2273 – 22

The denominator value is 9900.