Time and Distance Conversions and Ratios

In this class, We discuss Time and Distance Conversions and Ratios.

For Complete YouTube Video: Click Here

The first class was on time and distance. We discuss some formulas.

Speed = Distance / Time

The below diagram shows a distance D from location A to B.

Assume the distance is 180 km.

You traveled from point A to point B in 3 hours.

Speed = D/t = 180/3 = 60kmph.

Important: The graphical example below helps solve the problems and understand the formula.

Speed = Distance / Time.

The speed we are finding is indirectly average speed. How?

The below diagram shows the example to understand why the average speed?

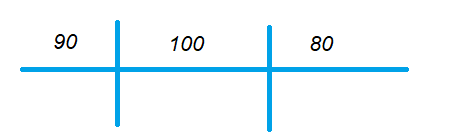
From point A to point B, the distance is D.

we traveled some distances at a speed of 90 kmph and others at a speed of 100kmph. The Remaining distance at a speed of 80kmph.

How do we find average speed?

Average speed = (90 + 100 + 80) / 3 = 90kmph

If we find the speed using Distance/Time, we get the value 90.

So indirectly, average speed.

No one will travel at a constant speed all the time. So speed is average speed.

Conversions:

Speed is measured in km/hr and m/s

km/hr = kilometers per hour

m/s = meters per second.

1 km/hr = 1000 meters / 60 * 60 second = 5/18 m/sec

x km/hr = (x * 5/18) m/sec

1 m/s = (1/1000 km)/ (1/60 *60 hr) = 18/5 km/hr

x m/sec = (x * 18/5)km/hr

Ratios:

If the ratio of speed of A and B is a: b then the ratio of times taken to cover a same distance is 1/a : 1/b or b : a

Important: The above statement please note of distance is same.

Speed = Distance /Time

Time = Distance / Speed

t1 = D/ s1 where s1 = a

t2 = D /s2 where s2 = b

t1 : t2 = D/s1 : D/s2

t1 : t2 = 1/a : 1/b

t1 : t2 = b : a