Expected Value or Mean of Probability Distribution

In this class, We discuss the Expected Value or Mean of Probability Distribution.

For Complete YouTube Video: Click Here

The reader should have prior knowledge of probability distribution. Click Here.

Expected Value or Mean:

Suppose a discrete random variable X assumes the values x1, x2, . . xn. and the corresponding probabilities p1, p2, . . pn.

Then the expectation of X or the expected value of X is denoted by µ or E(X) = Σi=1ton (xi pi). —–eq1

Important: Expected value = Arithmetic mean of the distribution. Why?

Arithmetic mean given frequencies = (Σi=1ton (xi fi))/Σi=1 to n(fi). —eq2

With example understand how eq1 and eq2 are equal.

Example: Toss three coins

Random variable X = Number of heads.

The below table shows the probability distribution for random variable X.

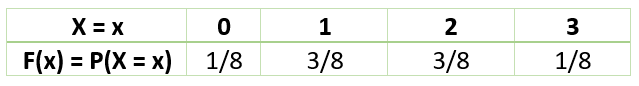
Expected value = Σi=1ton (xipi)

In place of frequencies, we are placing probability values.

Probability values are simply the frequency of occurring the event.

Probability or frequencies are both the same.

The denominator sum of all probabilities is equal to one.

E(X) = 0*(1/8) + 1*(3/8) + 2*(3/8) + 3*(1/8)

E(X) = 12/8 = 1.5

Next, class examples will help the reader when to use the expected value of probability distributions.