Variance and Game Favorable Example on Probability Distribution
In this class, We discuss Variance and Game Favorable Example on Probability Distribution.
For Complete YouTube Video: Click Here
The reader should have prior knowledge of the variance of a probability distribution. Click Here.
Example 1:
The below table shows the probability distribution for a random variable X.
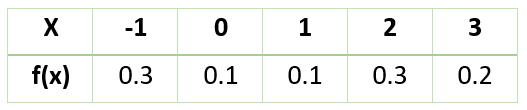
Find variance?
Solution:
E(X) = μ = all x Σ xf(x)
E(X) = -1(0.3) + 0(0.1) + 1(0.1) + 2(0.3) + 3(0.2)
E(X) = 1
Variance = : σ2 = E(X2) – (E(X))2
E(X2) = all x Σ x2f(x)
-12 0.3 + 0 (0.1) + 22 (0.3) + 1(0.1) + 32(0.2)
= 3.4
σ2 = E(X2) – (E(X))2
σ2 = 3.4 – 1
σ2 = 2.4
Example 2:
A player wins if he get 5 on the dice.
player looses if he gets 2 or 4 on the dice.
if the player wins heget 50.
If the player losses he get 10.
Otherwise, he has to pay 15.
Is the game favourable to the player?
Solution:
Random variable X = {-15, 10, 50}
P(X) = { 1/2, 1/3, 1/6}
Player will get 50 if he throws 5 on the dice.
P(X = 50 ) = 1/6
Similarly P(X = 10) = 1/3
P(X = -15) = 1/2
Find E(X) why?
If he plays the game repeatedly, The amount the player will get is the expected value.
E(X) = μ = all x Σ xf(x)
= -15(1/2) + 10(1/3) + 50(1/6)
= 25/6
= 4.2
The average amount player will get 4.2
The game is favourable to the player.
